Understanding the Finite Elements Method (FEM)
The resolution of the non-compliant structures, as well as the development of safe and modern engineering projects, entail the union between technical knowledge from the multiple technology-related disciplines available.
The usage of Finite Element Method (FEM), is presented as an option to ensure engineers capability of presenting assertive solutions to the stalemates which, disconnected to said method, would be excessively expensive, complex or of dubious reliability.
In other words, the use of FEM based on computational performance simulations is an interesting resource to ensure the quality and reliability of engineering projects.
To review basics FEM concepts read Understanding The Finite Element Method. In order to better embrace the meaning behind FEM, and its importance to modern engineering, continue reading this articles.
What is FEM?
The Finite Elements Method advocates, as its name implies, a problems’ domain division into smaller pieces that interact within themselves achieving the solution by differential equations.
This process, also known as discretization, enables the analysis of engineering issues as it simplifies the complex geometry of a wide range of objects and equipment dividing them into smaller pieces (as can be seen on Figure 1).
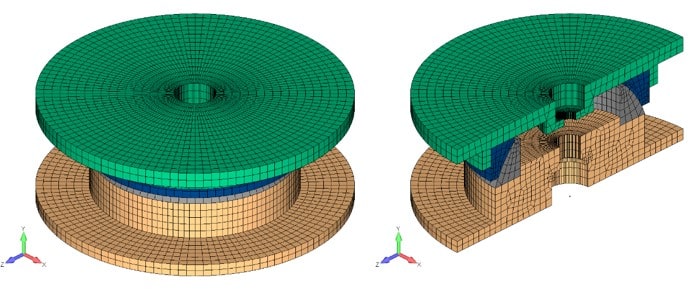
(Private archive from a KOT Engineering study case)
This approach allows that each one of the small elements, limited by outlines and known behaviors, represent a continuous dominium to the material being simulated.
It is thus important to emphasize that, according to the final aim of the analysis, the elements can portray different shapes, such as: triangles, squares,tetrahedron, pentagon, hexahedron or other rarer formats.

(Private archive, KOT Engenharia 2019).
Applying FEM
After the understanding of the more fundamental concepts, inquisitions of the relationship between the elements become necessary. Each of these elements is connected through the points known as knots. The combinations formed between the knots and the elements is, in turn, called a “mesh”. The precision of a FEM-based analysis will rely on the quantity of subdivisions adopted as the chosen will determine the size, quantity and the shape of the finite elements.
Such simplifications allow, in a practical manner, that the computational analysis could potentially be applied to each one of the small elements. Thus, it becomes possible to efficiently analyse the overall performance of a structure and components which present an, initially, complex behavior.
By solving problems using this technique, it is crucial to consider that: the smaller the elements, the bigger their quantity in a determined mesh chosen for the FEM analysis, and bigger the results precision. However, it should bear in mind that the number of elements directly influences the computational processing time. Depending on the structure’s characteristic to be analyzed and the distribution of the load, a certain limit for reducing the elements is reached. After this limit, the precision gain is negligible and disproportional to the associated computational cost.
Thereby, the acquaintance with the technique’s foundations allows the improved usage of software to carry out the finite elements analysis, also enabling an increasingly assertive, consistent and reliable interpretation through the results presented by them.
In order for the study to be developed, it is essential to focus on some key requirements which need to be defined before the computational modelling. It is important to have the detailed drawings of the structure which will be analysed at hand, also containing profile information, connections and the materials properties. In case this information is not promptly available, a field analysis must take place and the material duly tested.
To know more about how the Finite Method Analysis can assist you to solve structural problems:
Get in touch with KOT’s specialists team!

Rafael Brasil
Mechanical / Aeronautical Engineer from UFMG and post-graduated in Structural Engineering from the same university. He had the opportunity to work at the European Aeronautic Defense and Space Company (EADS), in Germany. He is currently Development Director at KOT Engenharia, leading dozens of projects related to the Structural Integrity of assets and equipments.










Leave a Reply